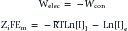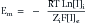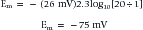Chapter 9 Electrodiagnostic Medicine I
Fundamental Principles
Action Potential Generation
Nerve and muscle tissues are capable of generating an electrical difference across their cellular membranes, and can generate and sustain a propagating action potential. Interestingly, action potential generation is relatively similar between these two dramatically different tissues. The reader is strongly encouraged to read basic physiology texts25,28 to gain a more complete appreciation of the intricate relationship between excitable cells and their associated action potentials.
Resting Membrane Potential
All living cells generate an electrical potential across their membranes, with the intracellular region relatively negative compared with the extracellular region.28 This potential difference across the cell membrane is referred to as the resting membrane potential. The development and maintenance of the resting membrane potential can be explained by a simple model.
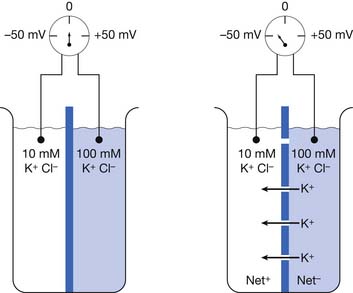
We can partition a beaker into right and left halves with an impermeable membrane containing multiple closed potassium channels. Assume this membrane separates two different concentrations of a potassium chloride (KCl) solution with a 10-mM concentration on one side and 100-mmol/L concentration on the other (Figure 9-1).17 Potassium chloride in solution exists as positive potassium (K+) ions (cations) and negative chloride (Cl–) ions (anions). A voltmeter (a device that detects potential differences) measuring the two solutions fails to detect a potential difference because there is a lack of physical continuity between the left and right halves of the beaker when the partition’s potassium channels are closed. In other words, the two solutions are electrically independent.
If we now open the membrane’s potassium channels, K+ cations will flow “down” their concentration gradient from the high (100 mm/L) to low (10 mm/L) ion concentration side of the beaker (see Figure 9-1). The potassium ions will continue this directional flow until there is a balance between (1) the forces of the physical concentration gradient difference driving potassium to the lower-concentration region, and (2) the electrical gradient opposing this directional ion flow. Because there are only potassium channels, the negative chloride ions cannot pass through the membrane and remain on their respective sides of the beaker. As more and more positive potassium ions leave one side of the beaker, there begins to develop an unbalanced or “excess” amount of negative charges (Cl–) on the high-concentration side of the beaker, with an equal buildup of “excess” positive charges (K+) on the other side of the beaker. The increasing net negative charge of the beaker half with the increasing number of unbalanced chloride ions begins to make it increasingly difficult for the positive potassium charges to leave the high-concentration side of the beaker. This is because the progressively building net negative charge increasingly attracts those remaining positive potassium ions. Similarly, a growing amount of positive potassium ions on the formerly low potassium concentration side of the beaker begin to increasingly repel additional potassium ions attempting to enter this side of the beaker.
The above simple example can be applied to all cells in the body, and in particular to nerve and muscle cells. A nerve’s axon will be used as a prototypical example, although the same principles apply to muscle cells. The nerve cell is known to have a specialized cell membrane permeable primarily to potassium and chloride ions in the resting state, because of proteinaceous transmembranous ion channels rendering the membrane selectively permeable (i.e., semipermeable).31 The potassium channels are referred to as passive, because they are always open and permit the free flow of this ion. Contained within the axon but incapable of crossing the cell membrane are large, negatively charged protein molecules. This negative charge attracts potassium ions from outside the cell to enter through the passive potassium channels, resulting in a buildup of potassium ions within the axon. This process continues until there is so much potassium within the axon that the continued entry of more potassium ions is prevented by the high intracellular potassium concentration, even though all the negative charges have not yet been electrically balanced. This is because the force of the accumulated potassium ions’ concentration gradient now attempting to drive potassium out of the cell is just large enough to balance the intracellular anions’ electrical force attracting potassium ions into the cell.
Nernst Equation
This formula can be rearranged to find the potential at which the electrical work just balances the concentration work (i.e., the dynamic equilibrium or resting membrane potential) by solving the above equation for Em
The above equation is more commonly known as the Nernst equation, and is a mathematical statement of the potential at which all the electrical and concentration forces are balanced in the cell’s resting state (i.e., the resting membrane potential).28,31 By substituting the actual values for the different variables and using the more familiar base 10 logarithm, the equation converts to the more recognizable form. Also, the approximate concentration ratio of intracellular to extracellular potassium is 20:1. The Nernst equation then becomes:
Sodium Pump
Experimentation has shown that the Nernst equation predicts the resting membrane potential quite well with different extracellular potassium concentrations, as long as the potassium concentration is relatively high. When the extracellular potassium concentration is low, there is a deviation of the resting membrane potential from that predicted, with a less-negative potential achieved. This finding suggests that another ion (sodium: Na+) has some influence on the resting membrane potential. It turns out that sodium has a very high extracellular concentration compared with intracellular ion concentration, which results in small quantities of sodium ions leaking into the membrane through a few passive sodium channels present in the cell’s membrane. This relative impermeability of positive sodium ions, combined with a high extracellular but low intracellular sodium ion concentration, and the resulting electrical drive to enter the cell (negative inside), would tend to “run down” the cell resting membrane potential over time. Fortunately, the cell has developed a mechanism whereby this run down is prevented. Located within the cell membrane is an energy-dependent sodium-potassium pump that pumps in potassium ions and pumps out sodium ions in just the right ratio to match the sodium ions entering and the compensatory potassium ions exiting the cell. Two sodium ions are extruded for every three potassium ions taken into the cell. The sodium-potassium pump maintains the exact ionic balance necessary to sustain a stable resting membrane potential by maintaining this 2:3 ratio.
Goldman-Hodgkin-Katz Equation
An important modification of the Nernst equation is the inclusion of ion permeability as being the primary influence on the cell’s transmembrane voltage. The greater an ion’s permeability, the more likely it is to influence the transmembrane potential. This is because the equilibrium potential of the most permeable ion in effect becomes the cell’s resting membrane potential. The equation accounting for the different permeability (designated as “p” in the equation below) factors is known as the Goldman-Hodgkin-Katz equation28,30,31:
Action Potential Generation
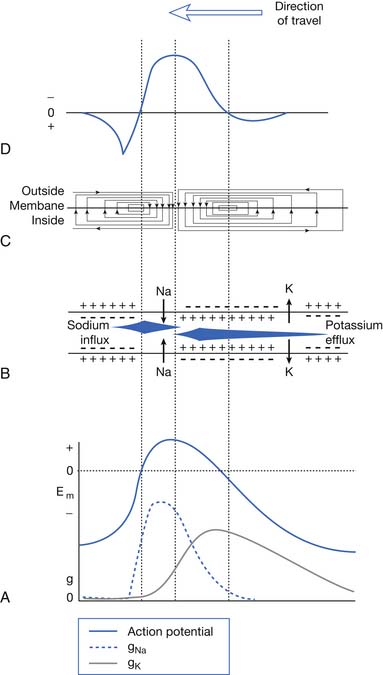
In addition to the passive (always open) potassium ion channels and relatively few passive sodium ion channels, there are also sodium and potassium ion channels within nerve and muscle cell membranes modulated by transmembrane voltage differences. These channels are voltage-gated because they open and close depending on the transmembrane voltage.25 Muscle and unmyelinated nerve contain both sodium and potassium voltage-gated channels. These voltage-gated sodium and potassium ion channels are closed at the resting membrane potential. If the transmembrane voltage changes toward a more depolarized direction (less negative) and reaches about 15 to 20 mV less negative than the resting membrane potential, the voltage-gated sodium channels open. This results in an increased permeability of the sodium ion, a process known as sodium activation. As noted above, the Goldman-Hodgkin-Katz equation predicts that the transmembrane potential shifts toward the sodium ion equilibrium potential. This massive shift in transmembrane potential is referred to as depolarization. After staying open for a short period, the sodium gates automatically close (sodium inactivation), with a return of the resting membrane potential again dictated by the resting state ion permeabilities (repolarization). In muscle and unmyelinated nerve membranes, a delayed opening of potassium voltage-gated channels occurs secondary to the depolarization and assists in repolarization.
Very few ions actually cross the membrane for sodium-induced depolarization or potassium-mediated repolarization. The region of membrane where there are large numbers of voltage-gated sodium channels in the open conformation acts as a so-called current sink for sodium ions to “sink,” or enter, the cell’s interior. This implies that a nearby source for the sodium ions must be present. The surrounding extracellular membrane region acts as the current source, thus permitting an ionic flow from the region surrounding the current sink. Sodium ions are thereby removed from the outside of the membrane surrounding the sink (making this region relatively more negative) and deposited on the inside of the cell (Figure 9-2). The newly introduced intracellular ions migrate to help neutralize some of the cell’s interior negative charges. This current flow, or charge transfer, from the extracellular to intracellular space is referred to as a local circuit current.17 The net effect of charge transfer is to make the cell’s interior less negative and exterior more negative, thereby shifting the transmembrane voltage in the more depolarized direction about the current sink. If the charge transfer is sufficient to depolarize the cell by approximately 15 to 20 mV, the membrane surrounding the sink is induced to permit sodium activation and hence undergo a significant depolarization. This process can then continue along the length of the cell.
The above process creates an action potential spike at the original site of sodium activation. A mechanical, chemical, or electrical stimulus that causes the membrane potential to reach threshold over a localized region is all that is required to serve as the initiating stimulus of action potential generation. Once the process begins, it is self-sustaining as long as there are sufficient ion channels to repeat the depolarization process. The intracellular action potential is essentially a monophasic positive spike: –75 mV resting potential; +40 mV spike; return to resting –75 mV with sodium inactivation and potassium activation (see Figure 9-2). The membrane’s threshold value must be reached to generate the self-sustaining action potential that is the same at all regions of the membrane. This concept is referred to as the all-or-none phenomenon.
The nodes of Ranvier in myelinated nerves lack voltage-gated potassium channels and contain only voltage-gated sodium channels.47,48 The action potential actually “jumps” from one node to the next, creating an efficient means of action potential propagation that is referred to as saltatory conduction. Repolarization in myelinated nerve does not require a delayed potassium current. As noted above, the resting membrane potential is restored once the permeability of sodium is reduced. Passive “back-leak” sodium and potassium currents are thought to mediate the discharge of the membrane’s capacitance, which accumulates over time with multiple action potential discharges.
Physiologic Factors Affecting Action Potential Propagation
Gender
Only a few studies have attempted to investigate the difference in nerve conduction studies between men and women.3 Noted is a slight increase in the antidromic sensory nerve amplitudes for both the median and ulnar nerves recorded from the digits in women. Also, women demonstrate a greater nerve conduction velocity (NCV) for upper and lower limb nerves compared with men. Both of these differences, however, are eliminated when one considers limb length and digit circumference (see below).40
Aging
Several generalizations can be made regarding peripherally evoked sensory nerve action potentials (SNAPs) and aging. The conduction velocity demonstrates a consistent decline approximating 1 to 2 meters per second per decade.36 The duration of SNAPs is about 10% to 15% longer in persons aged 40 to 60 years, and 20% longer in those aged 70 to 88 years, compared with persons aged 18 to 25 years.8 Compared with the 18- to 25-year-old group, the SNAP amplitude is one half and one third, respectively, for the 40- to 60-year-old and 70- to 88-year-old groups. The distal sensory latencies reveal a similar prolongation with age. There is a suggestion that the median and radial nerves do not demonstrate considerable alteration with age.19 At present, there is disagreement as to the magnitude of change in SNAP parameters induced by the aging process.
The results of aging on conduction velocity have been examined in a number of upper and lower limb nerves. Motor NCVs reveal similar changes to those described for sensory nerves. The newborn’s motor NCVs are about half of adult values, which are reached by 3 to 5 years of age.2 After the age of 50 years, the conduction velocity of the fastest motor fibers progressively declines by approximately 1 to 2 m/s per decade. There is a concurrent increase in the distal motor latency and decrease in the motor response’s amplitude with advancing age. H-reflex latency demonstrates little alteration with aging in the healthy elderly.20 The decrease in amplitude is difficult to ascertain clinically because of the wide range of normal H-reflex amplitudes and the central effects on amplitude that are difficult to control and quantify.
Digit Circumference
Females consistently demonstrate significantly higher antidromic SNAP amplitudes for the median and ulnar nerves recorded from the second and fifth digits.3 A negative linear correlation exists between finger circumference and amplitude for these two nerves. It is known that as the distance between the recording electrode and neural generator increases, the amplitude precipitously declines. Increasing the circumference of the finger displaces the electrode further from the nerve. Because men have significantly larger finger circumferences than women, this appears to explain the difference in SNAP amplitudes. There is no evidence that this difference is due to an intrinsic neural difference between male and female nerves.
Height
Several investigations have documented slower lower limb NCVs in taller compared with shorter individuals.9,33 This difference is independent of the limb’s temperature or subject’s age. The etiology of this finding is unknown. Distal nerve tapering or an abrupt change in axon diameter has been speculated to account for this finding, but the exact etiology has not been definitively identified.14,46
Temperature
Temperature is one of the most important factors influencing nerve conduction studies. As the temperature of the nerve is lowered, the amount of current required to generate an action potential increases. This decreased excitability is a direct temperature effect on the nerve’s action potential–generating mechanism at the nodes of Ranvier, and not a result of membrane resistance changes. The transmembrane resistance is not increased by a drop in temperature.26,27 In addition to excitability, the configuration of an action potential is profoundly affected by a drop in temperature.
The action potential’s amplitude, rise time, and fall time all increase as the nerve’s temperature declines. The time required for the action potential of a cold nerve to reach its peak depolarization from the resting membrane level increases approximately 33%.38 Similarly, the time necessary for the action potential to return to its resting level is also increased, but much more so than the rise time (69%). Because both the SNAP duration and the spike height increase, the area of the SNAP increases dramatically at lower temperatures.
The first detailed investigation of temperature effects on NCV in human nerves revealed an NCV to temperature correlation of 2.4 m/s per °C for median and ulnar motor conduction.24 With every 1° C drop in temperature, there was a 2.4 m/s decrease in the conduction velocity. Reductions in conduction velocity for upper limb motor nerve fibers have also been found to approximate a decrease of 4% or 5% per °C.10,29 Correction factors using subcutaneous and intramuscular readings are equally correct, but it is more convenient and less painful to use surface measurements.22
In the upper limb, the relationship between temperature and NCV has been investigated for the surface temperature range of 26° C to 33° C, measured at the midline of the distal wrist crease. Calculations reveal that for median motor and sensory nerves, NCV is altered 1.5 and 1.4 m/s per °C, respectively, while the distal latency for both nerves changes about 0.2 ms/°C.21–23 The ulnar nerve demonstrated motor and sensory temperature relationships of 2.1 and 1.6 m/s per °C, respectively, and a distal motor and sensory latency correlation of 0.2 ms/°C.
Waveform Configuration Generation
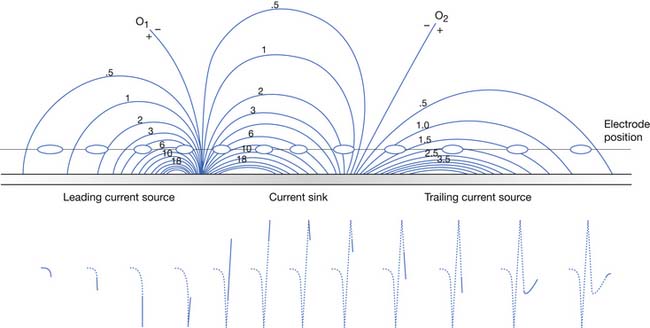
The current flow created by a depolarized region of membrane (current sink) is associated with a specific pattern of voltages known as isopotential lines (Figures 9-2 and 9-3).13 Recording a voltage at any point in space along an isopotential line results in the same voltage being recorded. As one moves further from the current sink, the corresponding voltage and the current density also decrease. The pattern of isopotential lines in space creates three distinct voltage regions. The current sink is associated with a negative voltage, while the two surrounding (leading and trailing) current sources are considered zones of relative positive voltage. Separating the current sink zone from the current sources are zero isopotential lines. These lines correspond to regions of zero voltage associated with that portion of the recorded waveform crossing the baseline (see Figure 9-3).
An action potential with its local circuit current and associated isopotential lines propagating past an electrode can be considered essentially equivalent to a stationary action potential sequentially sampled by a recording electrode passing through its electrical field (see Figure 9-3). The latter situation is used as an example for discussion purposes because it is easier to visualize the ensuing action potential waveform.
Suppose a propagating nerve or muscle action potential is frozen for an instant in time. A characteristic pattern of isopotential voltage lines is described in the region surrounding the nerve or muscle. A recording electrode can then be moved through the activated tissue’s electrical field to simulate a propagating action potential (see Figure 9-3). The final waveform configuration associated with an action potential propagating along a straight portion of nerve or muscle is a triphasic waveform with a large negative spike flanked by an initial large and subsequent small terminal positive phase. For discussion purposes, positive is denoted by a downward cathode ray tube (CRT) deflection, while an upward CRT deflection designates a net negative potential difference between the two recording electrodes. This example implies that for both nerve and muscle, when an action potential approaches, reaches, and then travels past a recording electrode, the fundamental waveform configuration is triphasic. These same simple principles can be applied to understand the generation of all waveforms observed during the electrodiagnostic medicine examination.
Nerve and Muscle Waveform Configurations and Characteristics
Nerve Potentials
Sensory Nerve Action Potentials
Clinical Recordings
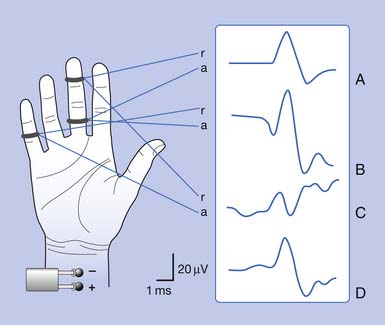
SNAPs can be obtained with either antidromic or orthodromic techniques.8 The term antidromic implies that the induced neural impulse propagates along the nerve in a direction opposite to its physiologic direction; for example, stimulating the median nerve at the wrist and recording the ensuing response from the second or third digit. A nerve will conduct an impulse proximally and distally from the point of stimulation by a depolarizing current. On the other hand, stimulating the median sensory fibers on the second digit and recording from the wrist is an example of an orthodromic technique. In orthodromic recordings, the sensory fiber impulses are detected at a site proximal to the stimulus as they travel physiologically from a distal to a more proximal region. Antidromically obtained SNAPs are usually larger and hence easier to assess, because the recording electrodes are in close proximity to the proper digital nerves than when a recording electrode is placed over the median nerve at the wrist for orthodromic techniques. Mixed nerve responses can have a component of both orthodromic and antidromic waveforms. For example, midpalm stimulations recorded at the wrist are a combination of orthodromic sensory and antidromic motor responses.
SNAP Configuration
Antidromic and orthodromic bipolar SNAP waveform recordings are typically biphasic rather than triphasic. The biphasic, negative-positive potential is a result of the bipolar recording technique and not a violation of volume conductor theory. Biphasic SNAP waveforms can best be understood by use of bipolar and referential recording montages for median nerve stimulation at the wrist (Figure 9-4).13 The median SNAP is indeed a triphasic waveform and conforms to the principles of volume conduction, but only appears biphasic because of the recording montage used.
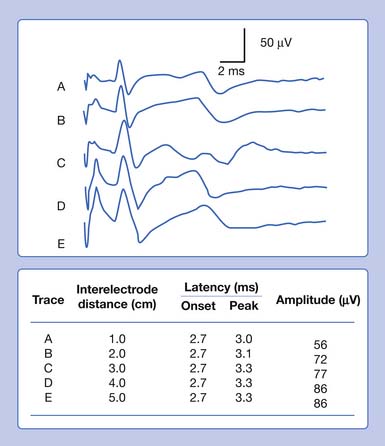
It is also possible to predict the optimal interelectrode separation to maximize the biphasic potential’s amplitude in the bipolar recording.17 The critical factor in this instance is the rise time, baseline to negative peak, of the biphasic potential, because it is this parameter that defines the waveform’s maximum amplitude. The recording electrodes must be located at a distance greater than the action potentials’ spatial extent, represented by the rise time duration. The rise time of most SNAPs approaches 0.8 ms, which represents a longitudinal extent of 40 mm for an action potential conducting at 50 m/s (50,000 mm/1000 ms = D/0.8 ms; D = 40 mm). If the two recording electrodes are separated by a distance less than 40 mm, some similar information regarding the main peaks of the waveforms recorded by both recording electrodes (active and reference) results in mutual cancellation of data, producing a potential with a smaller amplitude. A portion of the nerve will be depolarizing under the reference electrode while still in some degree of depolarization under the active electrode. At interelectrode separations greater then 40 mm, the biphasic potential’s negative peak amplitude will no longer grow, but the terminal positive phase will enlarge slightly and might change its configuration. These findings can be demonstrated by varying the distance between recording electrodes and observing the ensuing results (Figure 9-5). In effect, as the recording distance decreases below 40 mm, the amplitude of the recorded waveform declines and the peak latency shortens.
Muscle Potentials
Needle Insertional Activity
Normal Insertional Activity
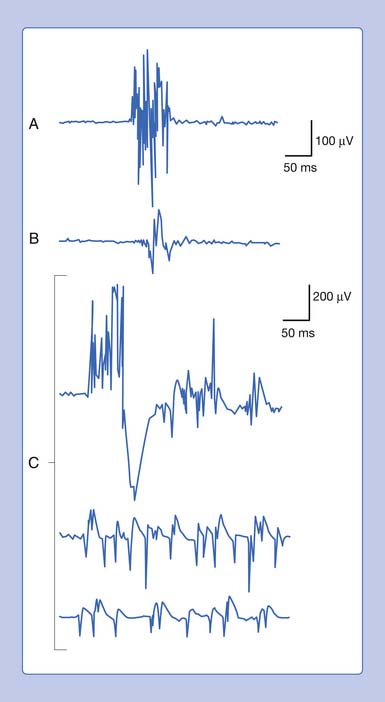
Placing a needle (monopolar or standard concentric) recording electrode into healthy muscle tissue and advancing it in quick but short intervals results in brief bursts of electrical potentials referred to as insertional activity (Figure 9-6, A).14 The observed electrical activity is believed to result from the needle electrode mechanically depolarizing the muscle fibers surrounding its leading edge as it pierces and deforms the tissue. Minimal and localized muscle tissue damage may occur from direct needle trauma and is the basis for the synonymous term of injury potentials. The purpose of including insertional activity analysis as part of the electromyographic examination is that the probing needle might provoke transient and/or sustained abnormal potentials associated with membrane instability before this abnormal activity with the muscle at rest.
< div class='tao-gold-member'>
Stay updated, free articles. Join our Telegram channel

Full access? Get Clinical Tree