Biomechanical Basis for Movement

1. Define and apply biomechanical concepts in the description of rudimentary movement patterns.

2. Discuss the difference between the kinematics and kinetics of movement.

3. Identify and discuss the kinematic principles as related to movement in a rehabilitation setting.

4. Discuss both the linear and angular kinematics and kinetics of movement, and explain how angular motion translates to linear movements.

5. Describe the differences among the different levers, and explain the concept of mechanical advantage as related to levers.

6. Describe Newton’s laws of motion.

7. Identify and discuss the different forces that act on objects and how the forces affect movement.

8. Discuss the concepts of mechanical loading, and describe how loading is associated with different types of injuries.

9. Discuss the principles of mechanical energy.

10. Describe the concept of equilibrium and identify the factors that contribute to stability.

Biomechanics is the study of the internal and external forces acting on the body and the effects these forces produce. The data obtained from biomechanical research provide a great deal of insight into human movement interactions in fields such as physical and occupational therapy, sports medicine, human factors, prosthetics, orthotics, and ergonomics.12,31 Clinical biomechanics, a subdiscipline, provides direct measures of human motion which influence our knowledge of injury mechanics, rehabilitation, treatment, and prevention. This information can directly affect how a physical therapist assistant (PTA) rehabilitates a patient, an orthopedist repairs a broken limb or ruptured ligament, an athletic trainer implements modalities for treatment, or a clinician evaluates an individual’s gait.^19,21,24

The field of biomechanics combines the study of applied human anatomy with that of mechanical physics. These combined sciences allow for detailed descriptions of how and why the human body moves the way it does and why a person may or may not have sustained an injury. Understanding the neuromuscular and mechanical factors associated with human movement provides the PTA with the knowledge and skills necessary for administering rehabilitative techniques correctly and performing patient assistive lifting tasks safely. These biomechanical descriptions influence health professionals to refine their knowledge and, therefore, their approach to injury rehabilitation as well as to consider new and innovative techniques that may lead to improved rehabilitation processes. This information also provides insight into the mechanical causes of injuries, potentially leading to safer participation as individuals interact with the environment.

Biomechanics can provide the health professional with a better understanding and a broadened knowledge of the causes and degrees of severity associated with an injury. In order to improve upon a current rehabilitative technique, repair procedure or treatment process, the mechanics associated with the causation of the injury must be fully understood. To help the health professional accomplish this task, several factors need consideration when determining the cause and severity of an injury ³⁶:

How much force was applied? (magnitude)

Where on the body was the force applied? (location)

Where was the force directed? (direction)

How long was the force applied? (duration)

How often was the force applied? (frequency)

Does the force application vary in magnitude? (variability)

How quickly is the force applied? (rate)

The major role of a PTA revolves around both the causes and effects of human motion and therefore it is imperative to have a fundamental understanding of the mechanical basis for whole body or segmental movement. Since biomechanics is concerned with the effects of forces on the human body, biomechanical principles are involved when a force is present. The following chapter will offer an overview of the science of biomechanics with practical applications for the PTA.

REFERENCE TERMINOLOGY

To facilitate the process of describing human movement, standardized terminology has been adopted to identify body positions and directions of motion (Box 16-1). Movements are defined based on a reference or starting position often referred to as the anatomic position (Fig. 16-1, A), which is a standing position with arms at one’s side and palms facing forward. From the anatomic position, three imaginary cardinal planes bisect the body along three dimensions (Fig. 16-1, B). The transverse or horizontal plane segments the body into upper and lower parts; the frontal or coronal plane separates the body into front and back parts; and the sagittal or anteroposterior plane divides the body into right and left parts. It is important to note that the planes do not necessarily divide the body into equal parts. However, when the segments are equal, the mid-intersection point of the transverse, frontal, and sagittal planes is referred to as the center of mass (COM) or center of gravity (COG). Although human movement is not restricted to a single plane, most named movements (e.g., flexion and abduction) are described based on the three cardinal planes.

BOX 16-1 Basic Directional Terms Used to Describe Body Parts or Other Objects in Relation to the Body

Anterior: Toward the front of the body

Posterior: Toward the back of the body

Deep: Toward the inside (core) of the body

Superficial: Toward the surface (skin) of the body

Distal: Away from the body or torso

Proximal: Closer to the body or torso

Inferior: Toward the feet

Superior: Toward the head

Lateral: Away from the midline of the body

Medial: Toward the midline of the body

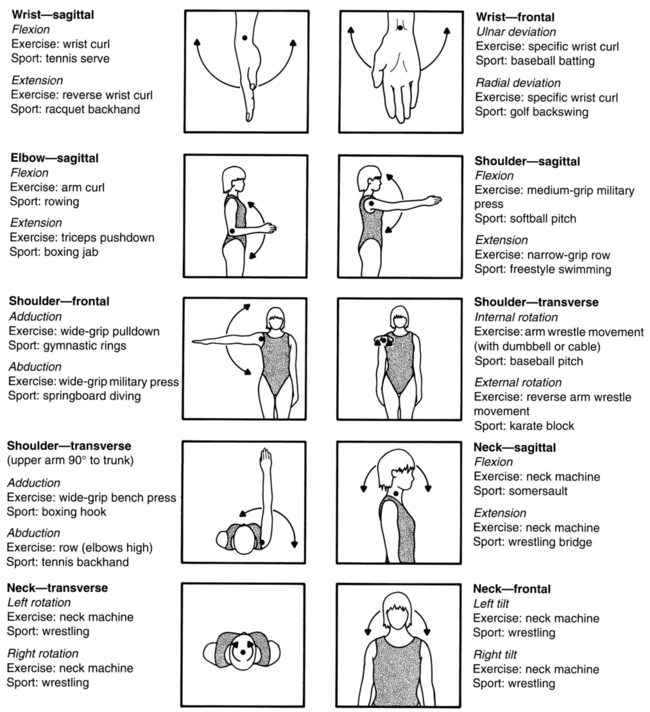
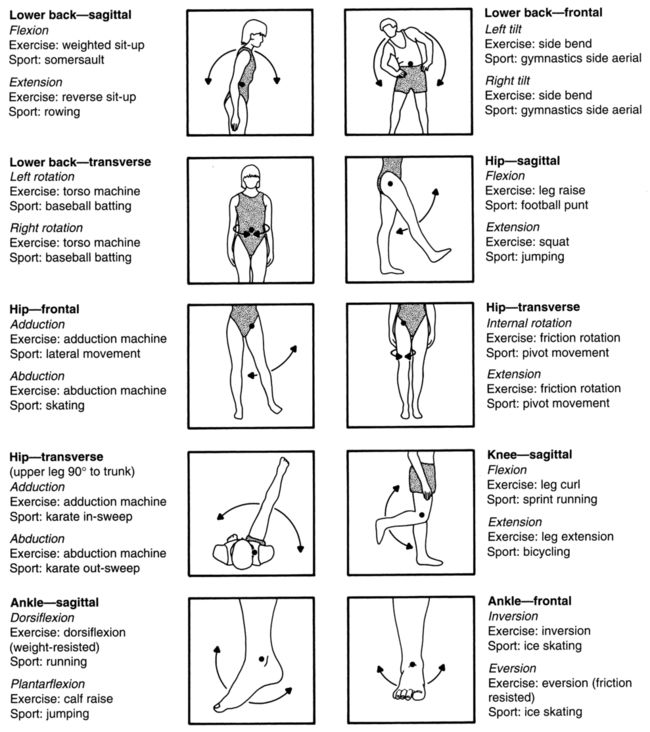
Movements in the transverse plane occur around the longitudinal axis, which runs superiorly–inferiorly while perpendicularly intersecting the transverse plane. These movements include medial and lateral rotation of the leg, thigh, and shoulder; supination and pronation of the forearm; and horizontal abduction and adduction of the shoulder. Movements in the frontal plane occur around the sagittal axis, which runs anteriorly–posteriorly while perpendicularly intersecting the frontal plane. These movements include abduction and adduction of the shoulder and hip, lateral flexion of the neck and trunk, elevation and depression of the shoulder girdle, and inversion and eversion of the foot. Movements in the sagittal plane occur around the frontal axis, which runs from left to right while perpendicularly intersecting the sagittal plane. These movements include flexion and extension of the knee, hip, trunk, elbow, shoulder, and neck; and dorsiflexion and plantar flexion of the foot as well as hyperextension movements. A key role of the PTA is to facilitate patient rehabilitation through the incorporation of various exercises using basic movement patterns. Therefore it is important to be familiar with the appropriate terminology for these movements (Fig. 16-2).

Fig. 16-2 Major movements of body segments. (With permission from *NSCA Journal* 14 (1) by Harman, Johnson, and Frykman, pp 47-54.)

BASIC CONCEPTS

To facilitate the discussion of biomechanical principles, a working understanding of various rudimentary concepts is essential. The following are definitions of some common terms along with their appropriate unit of measure. Most of these terms will be discussed in more detail as various biomechanical concepts are introduced.

Mass (m) is the amount of matter an object possesses within its physical boundaries; generally, the denser the material that comprises the object, the greater the mass. For example, muscle tissue is denser than fat tissue; therefore, two persons of equal size or volume may differ in mass if one is more muscular than the other.

Inertia is the resistance an object offers to a change in its state of motion (velocity) or direction of motion and is directly related to its mass. The greater the mass of the object, the more resistance it offers to any attempt at changing its velocity or direction of motion.

Force (F) is a push or pull acting on an object. A force will have both direction and magnitude, and it is commonly expressed in newtons (N). Forces applied to objects, if sufficient to overcome their inertia, will cause them to accelerate in direct proportion to the magnitude of the force.

Friction is created when two objects are in direct contact with one another and a force acts to impede motion of the objects. Frictional force can be increased or decreased by adding substances between the two surfaces, such as the installation of tennis balls on the rear support for walkers. Joint damage (osteoarthritis) caused by chronic exposure to high frictional forces can lead to arthroplasty.

COM or COG is the point within which the weight and mass of an object is equally distributed or balanced in all directions. The COM is important because when a force is applied to an object, the movement pattern will vary based on the relation of the point of force application to the COM.

Kinetic energy (KE) is energy by virtue of an object’s motion. The units for KE are typically expressed as a joule (J), however, one may also see units of newton-meters (N-m), which is an equivalent unit (i.e., 1 N-m = 1 J). An injury mechanism is predominantly due to the transfer of KE to the body arising from different sources under a variety of conditions: from blunt trauma (impact of object’s colliding with the body), penetrating trauma, acceleration/deceleration motion (rapidly moving forward and backward), and crushing weight (high compression forces).

Potential energy is energy generated by virtue of the position or shape of an object. Potential energy may be affected by how much elastic energy is generated by either stretching or compressing the object (e.g., cartilage, tendon, connective tissue) such that, if the distorting force is removed, the object will recoil to its resting length. The units for potential energy are typically expressed as a J; however, one may also use units of N-m.

Torque (T) is the product of the force and the perpendicular distance from the line of action to the axis point, also called lever arm length. Torque is considered a rotary force, but more specifically a measure of the ability of a force to cause rotation. Consequently, torque can be increased or decreased easily by altering the length of the moment arm of the force. A force couple is formed in situations where there are two torques that are equal in magnitude but opposite in direction. The resultant action of a force couple is rotation without any translation. Torque is typically expressed in units of N-m.

Work (W) is the product of force and the distance the object moves. If no displacement of the object occurs, even though force may have been applied to the object, no work was done. The unit of measure is a N-m or a J.

Power (P) is the rate of performing work, and can be expressed algebraically as the product of force and displacement over time. If work is accomplished very quickly (i.e., in a very short amount of time) then a higher magnitude of power is generated as compared to the same amount of work being done over a longer interval of time. Given this explanation, power is work divided by time. Power is expressed in watts (w), and 1 w is equal to 1 J of work per second.

Pressure (p) is a measure of the distribution of a force over a given area (force/area), and it is expressed in newtons per meter ² (N/m²). An example of the concept of pressure in a rehabilitation setting is the development of decubitus ulcers that commonly occur among diabetics.^3,32,38 Innovations in shoe design help dissipate the forces applied to the foot over a larger area (e.g., reduced pressure) during locomotion, thus minimizing soft-tissue damage and the incidence of ulceration.^6,10,35

Momentum is the product of mass and velocity used to determine the outcome of collisions between two objects of mass as well as to determine the ease with which one can stop or change the direction of travel when velocity is present. A motionless object has no momentum. The unit of measure is kilogram × meters/second (kg × m/sec).

Impulse is the product of the force magnitude and the force application time interval expressed in newton × seconds. The direct relationship between an applied force and the change in momentum it creates is known as the impulse-momentum principle. Consider a high force applied to the musculoskeletal system over a very short duration, as is often the case in force related injuries.