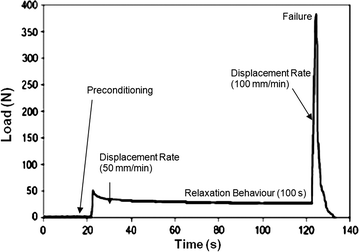
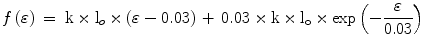Biomechanical Properties and Behaviours of the Wrist Joint

Fig. 2.1

The principal load behaviour of ligaments with respect to their elongation during constant elongation-rate [13–15]

There was also a report on the dependencies of these characterizing values on elongation rate which also consisted of the load relaxation behaviour over the course of time (Fig. 2.2) [14]. It was shown that after preconditioning at low loads, the specimens were elongated to 50 N at 50 mm/min. The specimens were held at a specific elongation, and the relaxation behaviour of the ligaments over a period of 100 s was obtained. After that, the specimens were elongated at 100 mm/min until failure. An issue was arisen as the presented results were problematic due to various measurement techniques applied. For instance, a study performed on the scapholunate ligament to investigate the slope of the quasi-linear region (related to ligament stiffness) used elongation rate of 50 mm/min [14], whereas an elongation rate of 100 mm/min was applied purposely in another study to determine the slope of various ligaments [16]. As the previous two studies were independent, another biomechanical study made the elongation rate to be dependent on the ligament length resulted in a rate ranging from 5 to 25 mm/s [17].

Fig. 2.2

The mechanical sequence for the scapholunate ligament [14]

Investigation on the effect of preconditioning routine has also been performed in previous studies. It was found that the preconditioning routine by stretching the ligaments enables averaging approach to the measurements [17–19]. This finding was proven to be logical as in the first ten load cycles, the restraint force of ligament for a given strain will decrease continually [19]. There was also a study with no preconditioning performed, which resulted in high variability of the results obtained [20]. Repeatable comparable data was one of the aims of these experiments. Out of these experimental studies, it was addressed that many difficulties faced due to the complexity of the carpal ligaments (the dimensions and shapes as well as the structures of the bones and articulations), and also the results obtained were incomparable with findings from knee ligaments experimental works [17–19].

Stiffness as one of the critical parameters has been addressed and its elastic modulus was found possible to be calculated once the physical dimensions of the ligaments were obtained. The stiffness of the extrinsic carpal ligaments has been discovered in previous works. It was found that the magnitude for the corresponding ligaments range from 10.9 to 46.4 N/mm [17]. It was also discovered that in neutral posture of the wrist, some of the ligaments were actually in tension [19].

Despite of the complex structure of the intrinsic ligaments which has thus far received little attention, their mechanical properties as found in several studies were profound and sufficient to be utilised in future studies. The stiffness of these intrinsic ligaments which range from 40 to 350 N/mm was obviously higher as compared to the extrinsic carpal ligaments. This difference was therefore showed that the mechanical properties of ligaments were highly dependent on its physiological location.

Overall, previous studies have progressively investigated the behaviour and properties of the ligaments associated with the wrist joint. A wide range of variability was reported, not only among different studies, but also among specimens within a given study comparing the same ligament. Despite these inconsistencies, the reported works significantly provide constructive fundamentals for specifying ligament behaviours, assisting better understanding on the static and kinematics of the wrist joint.

2.4 Current Trends in Biomechanical Modelling

Previous works had shown progress of modelling techniques, started with as simple as two-dimensional modelling with only a few bones involved, then progressively become more complicated and highly efficient attributed to the existing of high-end computational technology. However, due to the complexity of the wrist joint as compared to other joints, reported studies on the wrist modelling were still focused at the very early stage of its model development.

2.4.1 Rigid Body Spring Method

The patterns of load distributions, the kinematics, the effect of constraints as well as unique cases related to pathological conditions were aspects analysed in the previous studies [21–24]. Several approaches have been introduced to tackle aforementioned matters. In comparison with other available methods, rigid body spring method (RBSM) was commonly used to analyse multiple body contact forces as well as to perform kinematics analysis. This technique applied rigid body elements representing the bones, whereas the cartilages and ligaments were modelled using spring elements [24].

This method has also been utilised to investigate load transfer. In accordance to a two-dimensional study on 120 normal wrists, percentage of load transfer at the radiocarpal and ulnacarpal joints has been successfully unfolded [24]. 55 % of loads were transferred through radioscaphoid articulation, 35 % through radiolunate articulation whereas the remaining 10 % through the ulnacarpal articulation. Total load of 10 N simulating grasp action was applied at the five metacarpals. The cartilage with a stiffness of 15 MPa was simulated via compressive springs with stiffness of 200 N/mm each whereas tensile spring elements were used to model 28 ligaments. This study suggested that improvements should be taken into consideration in future studies especially regarding contact area between bones. A more recent study implemented three-dimensional RBSM wrist modelling sufficiently simulating the wrist joint kinematics and the findings were validated experimentally [25].

2.4.2 Finite Element Method

The finite element (FE) method is a computational numerical analysis technique, which is combined with computational modelling to obtain stress and strain patterns in modelled bodies, and has been widely used as solutions to engineering problems [26]. Variety of shapes and models can be analysed using FE method, either from as simple as a sphere, up to more complex and complicated design, such as an aeroplane. It can be defined as a tool used to predict behaviour of a complex problem, in which the structures are divided into smaller pieces so that complex mathematics formula can be occupied to calculate and simulate the required problems [27]. In other words, it uses a complex system of points called nodes which make a grid known as mesh. The mesh elements are connected to their adjacent nodes to react upon conditions assigned, including the material properties, the contact modelling as well as the boundary condition representing the real condition of that particular problem. This section has information on some aspects in FE analysis used to study the behaviours of the wrist, which covers types of mesh element and von Mises stress criterion. Information on findings and procedures used in previous studies on FE analyses were sufficiently provided in the following sections.

2.4.2.1 Mesh Element Types

There are two main types of element that have been widely used: tetrahedral and hexahedral. Tetrahedral with tetrahedron shape of elements can be either first order (with 4 nodes) or second order (with 10 nodes), and is preferable to model curvatures. Hexahedral, on the other hand, found to have better accuracy due to its nature of having relatively more degrees of freedom [28]. This brick shaped of element could also be categorized into first order (with 8 nodes) and second order (with 16 nodes) [29]. Despite of having greater accuracy, this type of element found to have less efficiency in performing contact analysis due to high number of nodes resulted in more tendency to distort. Therefore, tetrahedral was the better option for contact analysis.

2.4.2.2 Von Mises Yield Criterion

To analyse ductile material, it was often suggested to use von Mises stress which was formulated from a theory named the von Mises stress yield criterion [27]. This theory used to describe plasticity, and has been widely employed in material science and engineering field. Usually, prediction on yielding of material under any loading conditions was made based on this theory. The von Mises stress is calculated by combining stresses in the dimensions (x-, y– and z-directions) at any point on a structure, see Eq. (2.1). It was useful not only to determine the level of plastic deformity, but also to investigate the fatigue strength. Based on the theory, the material said to yield when the von Mises stress reaches the yield strength.

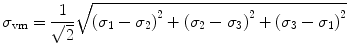
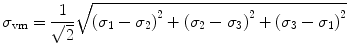
$\sigma_{\text{vm}} = \frac{1}{\sqrt 2} \sqrt {\left( {\sigma_{1} - \sigma_{2} } \right)^{2} + \left( {\sigma_{2} - \sigma_{3} } \right)^{2} + \left( {\sigma_{3} - \sigma_{1} } \right)^{2} }$

(2.1)

2.4.2.3 Past Finite Element Studies

The FE method has been widely used to study various human joints such as the hip and knee [30–39], and has been accepted by medical researchers as one of the important assistive tools in surgical planning [31, 32, 40–44]. Previous studies have shown its superior in varieties of analyses: pathomechanics, load transfer behaviour, contact analysis and also in investigation to assess viability of joints replacements including arthroplasty and arthrodesis. Although this method has been used for simulation of various human joints, the number of literatures related to the wrist was fairly limited with mostly reported on the model development [25, 44–48]. In the hip joint, only two bones were involved compared with 15 bones in the wrist joint, thus resulted in much more complicated analyses need to be done. Contact analysis of this multi-articulation joint proves to be especially tricky. In addition, the huge number of ligaments associated with the wrist joint make the analyses become much more challenging. For instance, wrong properties and locations of insertions resulted in unphysiological alterations of kinematics and dynamic of the joint. Additionally, solution convergence has been addressed as another main concern in finite element analysis of the wrist joint concerning the stability of the constructed FE models. Accurate and precise bone geometrical shape, articulations and the ligamentous structure are vital assuring successful analysis [46].

Therefore, of these few studies, there were lot of assumptions and simplifications made. For instance, the computational investigation of the wrist was started with two-dimensional FE analysis [49]. This study was performed to undertstand the behaviour of the Kienbock’s disease treated using ceramic lunate replacement method. In this study, the complexity of the joint was simplified by grouping bones into proximal, distal and forearm and excluding ligamentous constraint. It was quantified that contact analysis for the actual wrist joint could not be performed due to limited articulations. A more recent study on three-dimensional finite element analyses have been carried investigating static carpal load transfer [50]. This study sufficiently includes the whole wrist joint, consisted of separated 8 carpal bones, the radius and ulna which emphasised more at the radiocarpal joint. Non-physiological constraints were applied to achieve solution convergence. Similarly, studies by Cheng et al. [51] and Troy et al. [52] which partially analysed the behaviour of the wrist joint by having investigation on the distal radius fracture. To the best of the authors knowledge, there were none of previous studies successfully investigated the behaviour of the whole complete structure of the wrist joint without performing non-physiological constraint as well as wide range of parametric studies.

Regarding three-dimensional finite element studies on the pathological condition of the wrist, only a few numbers of works were found. There was a study performed to analyse the biomechanical effects of different position of Kirschner wires to provide initial stability of scaphoid fractures [48]. This study modelled the scaphoid whereas the adjacent bones were represented via articular cartilages with the thickness of 1 mm, assumed to be constant for each articulation. Another study on the biomechanical effect of the transverse carpal ligaments (TCL) release for surgically treated carpal tunnel syndrome (CTS) has been performed considering a complete model of the wrist joint. The TCL was constructed as a bundle of elements (not as springs which other studies used). It was found that due to the TCL release, the carpal bones at the radius side (the trapezium, trapezoid and scaphoid) displaced more towards radius during loading. This abnormal translation was doubt to be one of the implications of the postoperative surgery of the TCL release. Of these few studies on the diseased wrist joint, there was none made on the wrist joint affected by RA.

2.4.2.4 Ligaments Modelling

Tension-only spring elements were mainly used in the previous studies to simulate ligament. Despite the nature of the ligaments with nonlinear viscoelastic properties, due to simplification as well as limited resources, the issue on the linearity of the ligaments varied among previous studies. Linear properties of cables were used in a study by Ezquerro et al. [48] where different positions of Kirschner wires for scaphoid fracture fixation were analysed. However, most of them were used non-linear properties of springs to simulate the ligaments [25, 44, 46, 47]. The load-deflection behaviour discussed in Sect. 2.3 was used, governed by the superposition of a linear and exponential function:

$f\left( \varepsilon \right) \, = {\text{ k}} \times {\text{l}}_{o} \times \left( {\varepsilon - 0.03} \right) \, + \, 0.03 \times {\text{k}} \times {\text{l}}_{\text{o}} \times { \exp }\left( { - \frac{\varepsilon }{0.03}} \right)$